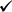Study Guide

Field 244: Multi-Subject: Secondary Teachers 
(Grade 7–Grade 12) 
Part Two: Mathematics

Recommendation for individuals using a screenreader: please set your punctuation settings to "most."

Sample Constructed-Response Item 1

Competency 0004 
Analysis, Synthesis, and Application

 start bold Use the information provided in the exhibits to complete the task that follows. end bold 

Using the data provided, prepare a response of approximately 400– to 600 words in which you:

• identify a significant mathematical strength related to the given standard that is demonstrated by the student, citing specific evidence from the exhibits to support your assessment;
• identify a significant area of need related to the standard that is demonstrated by the student, citing specific evidence from the exhibits to support your assessment; and
• describe an instructional intervention that builds on the student's strengths and that would help the student meet the area of need you identified above. Include a strategy for helping the student build a viable argument related to the given learning standard.

Exhibit 1: Background Information

A teacher is working with a student in a high school geometry class. The class is currently working on the following standard from the New York State Learning Standards for Mathematics.¹

 start bold Similarity, Right Triangles, and Trigonometry G-SRT  end bold 

 start bold Understand similarity in terms of similarity transformations end bold 

2. Given two figures, use the definition of similarity in terms of similarity transformations to decide if they are similar. Explain using similarity transformations the meaning of similarity for triangles as the equality of all corresponding pairs of angles and the proportionality of all corresponding pairs of sides. Note: With dilations or rotations, the center of the transformation must be specified.

In particular, the class is extending their knowledge of similar triangles to identifying other similar polygons. Previous work has included formal and informal definition of the concept of similarity as well as practice identifying similar triangles in a variety of orientations.

Exhibit 2: Student Work Sample

After a whole class review of what similarity means and how to identify it, students are working to identify which pairs of presented polygons are similar. The following exhibit shows three sample problems from the student's work along with the student's response to each question. Both the problems and the responses are representative of the student's work.

 start bold Worksheet Directions: Determine whether or not the two polygons are similar and explain your reasoning.  end bold 

Problem 1: 

Two quadrilaterals that visually appear to be rectangles are shown. Quadrilateral A B C D has a right angle at angle D, and has side A D of length 7 and side A B of length 8. Quadrilateral W X Y Z has a right angle at angle Z, and has side W Z of length 6 and side W X of length 7.

Similar, because they are both rectangles and there is the same relationship between the sides

Problem 2: 

Two quadrilaterals that visually appear to be rectangles are shown. Quadrilateral A B C D has a right angle at angle D, and has side A D of length 5 and side D C of length 2. Quadrilateral W X Y Z has a right angle at angle Z, and has side W Z of length 4 and side W X of length 10.

Not similar, because even though they are both rectangles, the sides are not related

Problem 3: 

Two quadrilaterals are shown. Quadrilateral A B C D has a right angle at angle D, a 100 degree angle at angle A, and a 45 degree angle at angle B. Quadrilateral W X Y Z has a right angle at angle x, a 100 degree angle at angle Y, and a 45 degree angle at angle Z.

Similar, because all the angles are the same, so the sides have to have the same relationship

Exhibit 3: Excerpt of Interview with Student

The following is an excerpt of an interview between the teacher and the student in which the teacher questions the student about the method of solution used to solve the problems shown in Exhibit 2.

Teacher:	How did you decide whether or not the two figures in Problem 1 were similar?
Student:	Well, I know that similar triangles have to have all the same angle measurements and that the sides have to be related to each other in the same way, so I think that must hold for these other shapes. So these ones are both rectangles, meaning that all their angles are the same, plus I see that if you add one to 7 you get 8 in the first rectangle and if you add one to 6 you get 7 in the second. That means they have the same relationship, so they must be similar.
Teacher:	What about in Problem 2?
Student:	Again, they are both rectangles, so all the angles are 90 degrees and the same. But when I look at the sides, even if I flip the second rectangle so it looks the same, I have to add three to get from 2 to 5 in the first but have to add six to get from 4 to 10 in the second. So they don't have the same relationship and can't be similar.
Teacher:	And how about Problem 3? Why did you say that they were similar?
Student:	This one was easy—all the angle measurements were the same, so even though there aren't any side measurements, the funny shape means that everything is going to match up and they must be similar.
Teacher:	Why does the shape have anything to do with it?
Student:	Well, like for the rectangles... you have to look at the sides because you can stretch a rectangle without changing the angles. For shapes like this, it must be like the triangles, where if you stretch a side, you would change an angle. So everything has to be stretched the same amount to keep the same angles, and that means that it's proportional.

Sample Strong Response to Constructed-Response Item 1

Sample Constructed-Response Item 2

Competency 0004 
Analysis, Synthesis, and Application

 start bold Use the information provided in the exhibits to complete the task that follows. end bold 

Using the data provided, prepare a response of approximately 400– to 600 words in which you:

• identify a significant mathematical strength related to the given standard that is demonstrated by the student, citing specific evidence from the exhibits to support your assessment;
• identify a significant area of need related to the standard that is demonstrated by the student, citing specific evidence from the exhibits to support your assessment; and
• describe an instructional intervention that builds on the student's strengths and that would help the student meet the area of need you identified above. Include a strategy for helping the student build a viable argument related to the given learning standard.

Exhibit 1: Background Information

A teacher is working with a student in a high school algebra class. The class is currently working on the following standard from the New York State Learning Standards for Mathematics.²

Reasoning with Equations and Inequalities AI-A.REI

Solve equations and inequalities in one variable.

4b. Solve quadratic equations by:

i) roman numeral 1  inspection, 
ii) roman numeral 2  taking square roots 
iii) roman numeral 3  factoring 
iv) roman numeral 4  completing the square, 
v) roman numeral 5  the quadratic formula, and 
vi) roman numeral 6  graphing.

Recognize when the process yields no real solutions.

In particular, the class is working on solving quadratic equations by factoring. Previous classes have covered the structure of expressions, writing expressions in equivalent form, and performing arithmetic with polynomials.

Exhibit 2: Student Work Sample

The student was asked to solve several quadratic equations by factoring. Shown below are two sample problems along with the student's response to each problem. Both the problems and the responses are representative of the student's work.

 start bold Worksheet Directions: Solve for x. Check your work and justify the steps you used to find the solution. end bold 

 start bold Problem 1: end bold  x² + 2x − 3 = 0x squared + 2 x minus 3 equals 0

x2 + 2x − 3 = 0 x squared plus 2 x minus 3 equals 0   factor this  (x )(x ) x times x  look at –3  negative 3  –3 • 1 = –3 negative 3 times 1 equals negative 3        3 • –1 = –3 3 times negtaive 1 equals negative 3   3 + –1 = 2 3 plus negative 1 equals 2   (x + 3)(x − 1) = 0 left paren x plus 3 right paren left paren x minus 1 rigth paren equals 0

(x + 3)(x − 1)  left paren x plus 3 right paren left paren x minus 1 right paren equals   = x • x + x • –1 + 3x + 3 • –1 equals x times x plus x times negative 1 plus 3 x times negative 1   = x2 − x + 3x − 3 equals x squared minus x plus 3 x minus 3

solve each of these for x  (x + 3) left paren x plus 3 right paren   x + 3 = 0 x plus 3 equals 0   −3  −3 start underline, minus 3, minus 3, end underline   x = –3 x equals negative 3   (x − 1) left paren x minus 1 right paren   (x − 1) = 0 left paren x minus 1 right paren equals 0   x − 1 = 0 x minus 1 equals 0  +1  +1 start underline, plus 1, plus 1, end underline   x = +1x equals positive 1

check answer  x = –3 x equals negative 3   x2 + 2x − 3 = 0 x squared plus 2 x minus 3 equals 0   (–3)2 + 2(–3) − 3 left paren negative 3 right paren squared plus 2 left paren negative 3 right paren minus 3   9 − 6 − 3 9 minus 6 minus 3  0 = 0  0 equals 0, checked  x2 + 2x − 3 = 0 x squared plus 2 x minus 3 equals 0   (+1)2 + 2(+1) − 3 = 0 left paren positive 1 rigth paren squared plus 2 left paren positive 1 right paren minus 3 equals 0   1 + 2 − 3 = 0 1 plus 2 minus 3 equals 0  0 = 0  0 equals 0, checked

You find the solution by solving the equation. Plug the answer back into the equation to check it.

Problem 2: x² + 6x = –8x squared plus 6 x equals negative 8

to factor this  take out an x  x2 + 6x = –8 x squared plus 6 x equals negative 8   (x)(x + 6) = –8 left paren x right paren left paren x plus 6 right paren equals negative 8	Now you can solve it.  x = –8  x equals negative 8  (x + 6) left paren x plus 6 right paren   x + 6 = –8 x plus 6 equals negative 8   6 = 6 6 equals 6  x = –2 x equals negative 2
check  x2 + 6x = 8x squared plus 6 x equals 8 gives you  (8)2 + 6(8)left paren 8 right paren squared plus 6 left paren 8 right paren which becomes  64 + 48 64 plus 48 then   112	x2 + 6xx squared plus 6 x gives you  (–2)2 + 6(–2)left paren negative 2 right paren squared plus 6 left paren negative 2 right paren gives you  4 + 124 plus 12 gives you  16

Since this is an equation, both sides must be equal, so you set them equal to each other and solve for x.

Exhibit 3: Excerpt of Interview with Student

The following is an excerpt of an interview between the teacher and the student in which the teacher questions the student about the method of solution used to solve the problems shown in Exhibit 2.

Teacher:	We will start with Problem 1. Can you tell me what you did to solve this equation?
Student:	First I wrote down two sets of parentheses. Then I factored it by looking for two numbers that when multiplied give 3, no, minus 3, and when you add them you get 2. I wrote out possible answers and found that 3 and minus 1 worked. Then I put them in with the x's like here (points to the product of two binomials set equal to zero).
Teacher:	It looks like after you factored the trinomial into two binomials, you set each of them equal to zero and solved the resulting equation. Can you tell me why you took that step? Can you justify why you set each binomial equal to zero?
Student:	Whatever is on the left side of the equation has to equal what's on the right side. So (x + 3)left paren x plus 3 right paren has to equal zero and so does (x − 1)left paren x minus 1 right paren, because equations have to balance. Then you just add or subtract the number to find the answer.
Teacher:	Let's look at Problem 2. Can you tell me what strategy you used to solve the equation?
Student:	I solved it just like I solved the first one. This time, though, it was easier to solve because it's easier to factor. You just take out an x.
Teacher:	After you factored the left side of the equation, what did you do next?
Student:	I set both sides of the equation equal to 8 and solved for x.
Teacher:	Can you justify why you took that step?
Student:	Both sides of an equation must be equal. So whatever is on the left side has to equal what is on the right side. So I set them equal to each other and solved for x.

Sample Strong Response to Constructed-Response Item 2

Performance Characteristics for a Constructed-Response Item

The following characteristics guide the scoring of the response to a constructed-response item.

Completeness	The degree to which the response addresses all parts of the assignment
Accuracy	The degree to which the response demonstrates the relevant knowledge and skills accurately and effectively
Depth of Support	The degree to which the response provides appropriate examples and details that demonstrate sound reasoning

Score Scale for a Constructed-Response Item

A score will be assigned to the response to a constructed-response item according to the following score scale.

Score Point	Score Point Description
4	The "4" response reflects a thorough command of the relevant knowledge and skills: The response thoroughly addresses all parts of the assignment. The response demonstrates the relevant knowledge and skills with thorough accuracy and effectiveness. The response is well supported by relevant examples and details and thoroughly demonstrates sound reasoning.
3	The "3" response reflects a general command of the relevant knowledge and skills: The response generally addresses all parts of the assignment. The response demonstrates the relevant knowledge and skills with general accuracy and effectiveness. The response is generally supported by some examples and/or details and generally demonstrates sound reasoning.
2	The "2" response reflects a partial command of the relevant knowledge and skills: The response addresses all parts of the assignment, but most only partially; or some parts are not addressed at all. The response demonstrates the relevant knowledge and skills with partial accuracy and effectiveness. The response is partially supported by some examples and/or details or demonstrates flawed reasoning.
1	The "1" response reflects little or no command of the relevant knowledge and skills: The response minimally addresses the assignment. The response demonstrates the relevant knowledge and skills with minimum accuracy and effectiveness. The response is minimally supported or demonstrates significantly flawed reasoning.
U	The response is unscorable because it is unrelated to the assigned topic or off task, unreadable, written in a language other than English or contains an insufficient amount of original work to score.
B	No response.

---

Acknowledgments

¹From the New York State Education Department. New York State Next Generation Mathematics Learning Standards. Internet. Available from http://www.nysed.gov/curriculum-instruction/new-york-state-next-generation-mathematics-learning-standards; accessed 1/8/2019.

²Ibid.

Copyright © 2019 by the New York State Education Department